It's time to lay it all down. I have been talking for a long time about
refractions in opposite directions in subjective prismatic experiments,
about colours that do not refract at all, I have even claimed that these
prismatic events happen in objective experiments too. So now is time to put
all those issues out here, in the open. Let's thus get moving straight into
the matter.
It is surprisingly easy to demonstrate that in subjective experiments the
colours R and B refract in opposite directions in a prism. To all intents
and purposes, it suffices to look through a prism (oriented as always with
the apex pointing to the left of the observer) at a picture like the one
shown below.
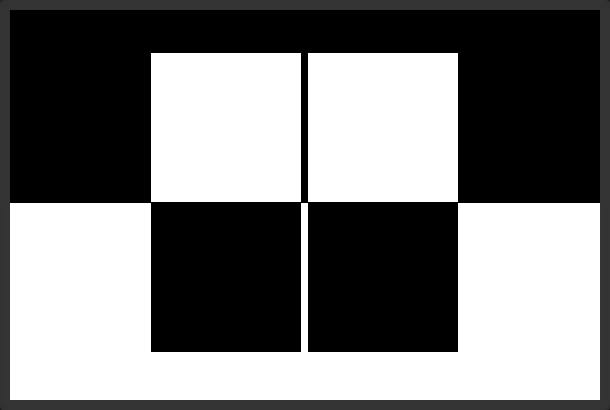
We are quite familiar with this kind of picture, for it has been used to
describe subjective prismatic experiments for a very long time. You may be
inclined to believe that this picture is in fact so well known that it does
not hold any secrets from us, and you could probably draw all the colours
that would be seen in a prismatic observation without having to actually
look through a prism. Well, you may know all those things, but I bet that
you didn't know that this picture shows quite clearly not only that R and B
refract in opposite directions, but also that G does not refract at all. See
image below.

This is what the observer will see from a distance of about 0.5 m. This
is one picture that brings to the attention of the observer not only the
three Newtonian primary colours, but also the Goethean trio of the
complementary colours. And it does even more than that. It also
demonstrates the perfect symmetry between those two sets of colours. To my
mind, however, the most important thing that it brings to attention is the
manner in which the colours are affected when observed through a
prism.
We know that the G bar is actually occupying the same space where the white
bar was in the original picture. The former white bar appeared white because
the three colours were superposed (in the space occupied by the G bar)
before they were refracted by the prism. It is clear therefore that in
subjective prismatic experiments R is refracted towards the base of the
prism, B in the opposite direction, towards the apex, and G is not refracted
at all. In fact, the R and B refraction in opposite directions is also
evident from the displays of the RY and BC combinations.
I want to show you a beautiful exposition of the subject we're discussing
now. This example comes from the pages of Dr. Pehr Sallstrom, who has
dedicated the best part of his work to promoting Goethe's theory of colours
to a status equal to that of Newton's. See image below.
(Click on the image to see it enlarged.)
The image is self-explanatory. At 1 there are four bars aligned vertically.
One of those bars is white, the others are coloured Red, Green and Blue. Then, at 2 there is
the image at 1 seen through a prism oriented with the apex to the left. At
the top, the white bar has produced the Newtonian spectrum. Below that Red has been deflected towards the base of the prism, Green has not been refracted
at all, and Blue was refracted towards the apex of the prism.
Now, if this is not conclusive evidence that the spectral colours are
behaving in subjective prismatic experiments exactly as I claimed, I cannot
imagine what kind of evidence would be more conclusive. And yet, Sallstrom,
a professional physicist with extensive expertise in optical phenomena and
colour theory, describes the outcome of that experiment by saying
nothing more than that "the three colours find their respective appropriate
positions"! It is absolutely incredible that he appears to be totally
oblivious to the fact that in order for the three colours to have found
their "respective appropriate positions" they must have obviously violated
the Newtonian rules of refraction! And that's not all either. On top of all
that, Sallstrom also fails to mention that in order for the three colours to
find their so-called "respective appropriate positions" it is absolutely
imperative that the observation is conducted through a prism oriented with
the apex pointing to the left of the observer! To merely say, as he does,
that the three colours find their "respective appropriate positions" when
"looked at through a glass prism" is a completely unacceptable faux
pas. That's clearly because one can look through a glass prism at the
same experiment and see an inverse distribution of the three Newtonian
colours!

I have always asked myself why apparently no one has noticed such blatant
discrepancies between the theoretical understanding and the experimental
evidence. It's hard to say, but I think I may have an idea. After all I
had also wondered why everybody, from Goethe onwards, described so clumsily how the
spectral bands that are seen in subjective prismatic experiments depend on
how the white and black areas are positioned relative to each other. ('If
white is above and black is below, and the prism is positioned with the
refractive angle...'etc.) To my mind it has always been plain and simple: In
subjective experiments the Blue-Cyan bands appear towards the apex of the
prism, and the Red-Yellow combination towards the base. Forget about the black background,
for it plays no active role in the process of spectra generation.
Black means absence of light, and it therefore cannot generate a spectrum.
Black, however, plays a very important passive role in prismatic
phenomena. I will elaborate on this topic in due time.

It is profoundly disturbing to see how grievously defective the conventional understanding of the prismatic
phenomena still is 350 years after Newton. Our physicists' only 'accomplishment' in all this
time has been to successfully supress any kind of potential dissent from the
mainstream fundamentalist dogma by adding more and sillier epicyclic
concoctions to it. Take, as a concrete example,
this page
from a website created by a former Professor Emeritus of physics at one of
the greatest German Universities there is. The page I'm referring to is
called Simple observations with a prism, and in a rather typical note
it begins with the following statements:
Little is needed for the following experiments: In addition to a prism
only a piece of black paper and white and coloured paper
strips. The following photographs objectify subjective observations. On the left
hand side the object is shown, to the right its appearance if viewed at
through a glass prism.
Please pay close attention to the statement contained in the second
sentence of the above paragraph: "The following photographs objectify
subjective observations". With this declaration in mind in a few moments I
will not only show you how plainly false that statement is, but also how
incredibly stupid the arguments used by this former Professor Emeritus on
that page truly are. Before getting to that though let me mention the first
monumental stupidity the Professor has already uttered to this point.
Apparently, Herr Professor is not even aware of the fact that--according to
the mainstream understanding itself--photographs simply cannot objectify
any subjective observation! That's because what the naked eye sees
through a prism is always exactly the same to what a camera will record,
when used in the same circumstances! Otherwise, if photos could indeed
objectify subjective observations our mainstream establishment would be
forced to accept that no subjective prismatic observations exist! Think
about that, Herr Professor.
Let us now examine the first experiment presented, which is thus described
by the author:
Put a thin strip of white paper on a dark surface and look at it through a
prism. You have to look in a different direction, and due to the differences
in the refractive index for the different wavelengths of light, the strip
now looks coloured. If the strip is sufficiently narrow, there are
essentially only three colours to be seen: red, green and blue-violet. This
is a special case of the phenomenon known since the 19th century under the
name of Bezold-Brücke shift.
Thus we perceive the spectrum of white light, if it is rather dim, as
made up of only three bands, red, green, and violet-blue, with hardly
perceivable transitions.
For the photographs, the arrangement was as shown in the adjacent
sketches, only the eye was replaced by the camera.
(Those "adjacent sketches" are shown below.)
Three rays of light from the white stripe are shown. The prism splits
them up because the higher the frequency, the more the light waves are
refracted. Only three refracted rays are drawn each time, but in reality
each ray creates a fan of rays. (The drawing is strongly
exaggerated.)
It is easier and clearer to draw only rays that reach the eye, if you
want to visualize the geometry (lower picture). The "white" rays are
indeed superpositions of rays of all the wavelengths present in the light,
and if only the portion ultimately reaching the eye is drawn, it should
not be concluded from this that the rest is not there.
Now, before anything else I must say that everything stated about this
experiment is such a cacophonous train of arguments that it is pretty
much impossible to make any sense of what it all means. And you don't have to
take my word for it, for in just a few moments you shall be able to judge for
yourself if what I said is true or not. In the meantime, I shall try my best to
discuss and clarify every single argument that is involved and relevant to the
greater picture of the subject concerned.
Let me begin with the spectrum that was apparently generated by the thin white
line that was observed through a triangular prism, which is shown in the
second picture of the first couple of images above. The plain reality is that
a thin white line as the one that it is purportedly observed subjectively in
this experiment will never be able to generate a spectrum like the one shown
above. For one very simple and straightforward reason.
In order for a thin line like that on display to be able to generate a
spectrum with three coloured bands as wide as those of the shown spectrum, the
observation would have to be conducted from a significant distance away from
the white strip. (I would say from a distance of about 1.5-2 m away.) This
causes a great problem, for the simple fact that a subjective prismatic
observation of a thin line (like the one in this experiment) will result in a
spectrum in which the B component will appear completely separated from the
other two colours. In fact, from such a distance even the R component would be
separated a little from the G one. And you can easily verify whether what I am
saying is true or not. All you have to do, of course, is look through your own
prism at the thin white strip from some distance away. You don't even have to
do it from the distances I had suggested. It suffices to conduct the
observation from about 80 cm to 1 m away, and you'll be able to see exactly
what I said.
The only way to conduct a subjective observation on the thin strip of this
experiment and obtain a spectrum in which the three colours will be right next
to each other is to conduct the observation from a distance of only about 20
cm away from the object under observation. The only problem is then that the
widths of the three colours would be about equal to the width of the white
source.
Now, if the first couple of pictures were ridden with errors (as well as with
quite a bit of disingenuity, I believe) the next two images are downright
perplexing in their fantasist display. What on earth they are supposed to
illustrate could only be perhaps described in some sort of gibber, and
gibberish is not something that I could claim to understand. Nonetheless,
there are enough clues encapsulated in their display to give me plenty of
opportunities to assess whether they carry any worthiness within, or rather
just a heap of caca.
The first thing to notice is that the prisms in the two illustrations are
depicted in positions of minimum deviation. This is a typical conventional
trait, which it is relatively useful in objective experiments but absolutely
useless in subjective ones. Now I'm pretty sure that this particular topic is
likely to create quite a stir amongst the majority of you, so I will take a
bit of time to discuss it in some detail.
The fact that most (perhaps all) conventional physicists use the position of
minimum deviation in both objective and subjective prismatic experiments is
one of the most enduring legacies that Newton has left to the world.
-------------------------------------------------------------------
I have just realised a few moments ago that basically every single subject
I had planned to discuss in this post I had already done so in many of my
old postings of the past. In fact, after reading most of my posts from the
past--for the first time, since I had published them--I was greatly
surprised (and quite pleased) at the amount, and depth, of the issues I had
already discussed in this blog. That realisation made me suddenly come to
yet another brand-new decision. (I am saying that because I had made similar
decisions in the past, as some of you surely know.)
Firstly, that from this point on I will make an earnest effort to avoid
discussing topics that I had already covered in the past. Secondly, that I
will genuinely push myself to periodically review the writings I had done in
the past (even though I completely hate the mere thought of having to do that). Thirdly, that from this point onwards I will only focus
my writing on my latest and most relevant work that I will consider worthy
of sharing with anybody else. Fourthly, that I will only make one final
reference to what we were discussing right before my drawing of this new
line on the sand, by using just a one-word sentence: Caca.
----------------------------------------------------
Of all the prisms I own one has always been my favourite. It is a quite
large (5 cm each side) equilateral prism, with a very high (in excess of
1.8) index of refraction. It was most likely made out of highly fused flint
glass. I have been using this prism for more than ten years, in both
objective and subjective experiments. I have placed this prism in positions
of minimum deviation many a time, and used it to conduct both objective and
subjective observations from that position. Many of the experiments I
conducted over the years I have also filmed. Indeed, a couple of them I have
posted on YouTube (see
this video, and
this video, for example).
A few years ago, however, I came to the conclusion that in subjective
experiments one should never conduct an observation through a prism placed
at minimum deviation. That conclusion was based on a number of reasons, of
which two were perhaps the most important. The first of those two reasons
was closely linked to the fact that unlike to the objective experiments (in
which minimum deviation came with a definitive list of advantages) in
subjective experiments minimum deviation came with not only a basically
empty list, but in fact with a number of distinct potential of
liabilities.
My conclusion also suggested that I should be able to determine and predict
how and where exactly I should place my prism in order to get and extract
the most eloquent set of data from a vantage point that is determined not by
the particular index of refraction of the particular prism involved but by its particular geometry. And in a relatively short span of time, I was able to discover everything I needed to obtain all the desired particulars of a subjective observation without any reliance on a setup of minimum deviation. To that end, I will next share with you all the experimental evidence that is required to prove the validity of my claims.
Suppose that you'd like to find out if there is a precise, mathematical process (rather than a laborious and ineffective one of trial-and-error) that would enable one to determine how to conduct a subjective prismatic observation of some object randomly placed anywhere in space. See the picture below.
That was the exact question I had asked myself a few years ago, and to a great degree of genuine surprise I was to find myself, after a relatively short period of investigation, on a straightforward path to a definitive answer. With a great deal of pleasure, I was to discover that the answer in question turned out to be not only comprehensive and simple, but also insightful and coherently beautiful. To see what I mean just imagine yourself at this point as a nosey neighbour that is looking through his window at the people that are walking on the street that is facing that particular window. Imagine next that the window in question is in your attic and that it is tilted at a 60० angle, just like the face of an equilateral prism. Now, shouldn't it be obvious therefore that the best perspective that such a window could offer would necessarily be running along the plane most perpendicular to the plane of the window itself?
Anyway, a handful of days later I was absolutely certain that what had begun as mere speculation had quickly evolved into a comprehensive array of scientific fact. In less than a week I had thus learned how to assess and conduct basically any kind of subjective observation, with such a high degree of precision that it practically enabled me to almost instantly determine, choose and predict in advance what, how, and where the eventual results should become reality.
So, let me show you how easy it is to determine where exactly an observer should first place his prism relative to the object under observation, and, secondly, where then he ought to place either his naked eye or the eye of a camera, in order to be sure of getting the best possible results of a subjective observation. Take a look at the picture below.
As you can see, we have simply drawn a line that is running perpendicularly towards the face of the prism through which the observation will be conducted. We have also decided at which particular point that perpendicular line should meet the face of the prism, and it is very important to realise that that particular decision had been made completely independent of any refractive conditions. (As it can clearly be seen, the line we had drawn is not at all affected by its travelling through the prism.)
But let us show you one more perspective of the setup involved in this experiment, before proceeding to the actual act of observation. The perspective this time was recorded at an eye-level view.
And now is time to see what kind of results our experiment yielded. See the two pictures below, and don't forget that if you click on them, you'll be offered enlarged views of the pictures.
So, the results shown thus far are most eloquent demonstrations about the validity of my work in the matter. Nonetheless, there is still a great deal of additional evidence needed in order to ultimately prove beyond the shadow of a doubt that everything I have been saying can comprehensively account for all--past, present and future--prismatic observations.
We'll return to the presentation of more prismatic observations in a few moments, but at this point in time I'd like to continue elaborating on a subject I had briefly touched on a little earlier into our current journey. Specifically, you remember my wondering if there was some mathematical method that one could perhaps discover and use as a highly efficient tool in all conceivable prismatic investigations, before arriving at the "perpendicular" possibility that we have been using thus far. As satisfying as that particular method proved to be I can tell you that it pales in comparison to the joy I felt a little later down the track, when I had learned that indeed there was an exact, mathematical way of determining where a prism should be placed relative to an object.
To cut a long story short I will simply say that one day it suddenly dawned on me that by using the geometry of two perpendicular planes that are intersecting at some point in order to exchange personal gifts, we had in fact been led to that spot by following two very precise and directly connected trigonometrical routes. To make our task easier to visualise, and in effect to thus get a better grasp of it in the end, I will now drop below an illustration I made. Let's have a look at it.
Consider an object (green circle denoted O) located at no particular place somewhere in space. An observer who, like us, had learned from experience how to determine what would be the ideal location where he should place his equilateral prism and conduct a subjective observation of O from, had drawn a line (h) which is running directly perpendicular from the object towards the face of the prism through which he will look at his target.
At this point he realises that although he had chosen this particular location based solely on a line of reasoning that relied on the perpendicularity of Cartesian planes, he could have also used basic trigonometry to arrive at the same conclusion.
Now, if you wonder what the point of this exercise was, I would like to draw your attention to the highlighted remark in the paragraph below (which you might remember from the page we had discussed earlier).
Put a thin strip of white paper on a dark surface and look at it through a prism. You have to look in a different direction, and due to the differences in the refractive index for the different wavelengths of light, the strip now looks coloured.
By all scientific principles, that should be rightfully deemed as one dumbfounding remark. In the context of the sheer reality out there, however, it becomes a real gem. It becomes a gem because in its elusiveness it is encapsulated the prevalent quagmire in the conventional understanding of prismatic phenomena. With this in mind let us return to the presentation of more subjective observations.
In the two pictures above, we have a subjective observation conducted with a water prism, photographed from two different perspectives. The index of refraction in water (1.33) is much lower than the index of refraction in the prism we used in the previous observations (1.879936). Yet, as you can see, the same principles led to the same outcome in both observations.
In the two pictures above, we used a glass prism with an index of refraction of 1.54.
For the observation captured in these two pictures we used a wedge prism whose angle at its apex is 30⁰. Effectively that is therefore one half of an equilateral prism. For this observation we firstly placed the prism with the tilted face fronting the camera, and then we changed its orientation with the straight face in its place. See the pictures below.